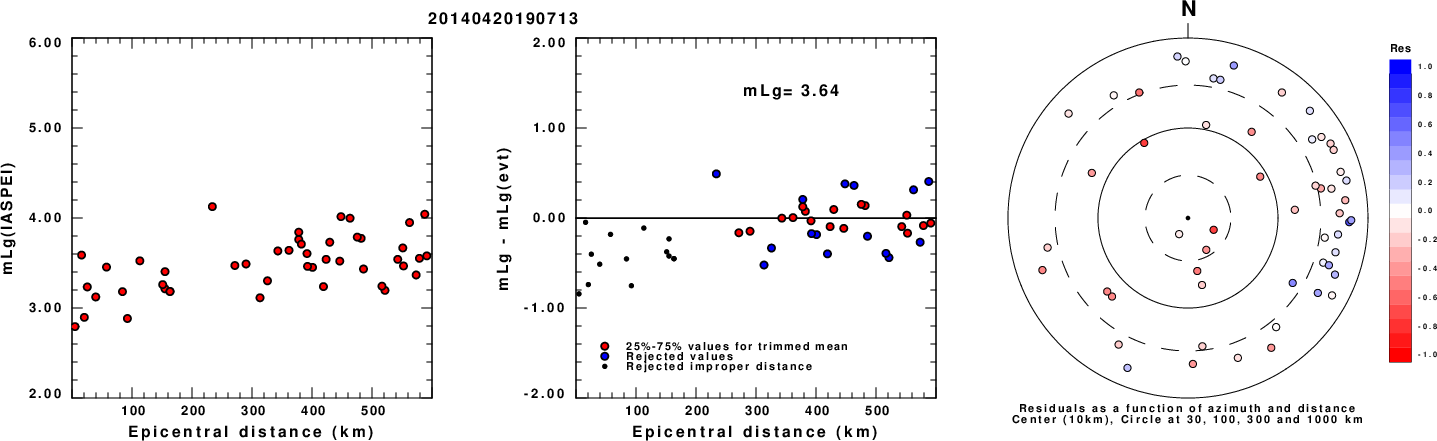
Left: mLg computed using the IASPEI formula. Center: mLg residuals versus epicentral distance ; the values used for the trimmed mean magnitude estimate are indicated. Right: residuals as a function of distance and azimuth.
The ANSS event ID is usb000ps3d and the event page is at https://earthquake.usgs.gov/earthquakes/eventpage/usb000ps3d/executive.
2014/04/20 19:07:13 35.774 -97.482 6.7 3.7 Oklahoma
USGS/SLU Moment Tensor Solution
ENS 2014/04/20 19:07:13:0 35.77 -97.48 6.7 3.7 Oklahoma
Stations used:
GS.OK025 GS.OK026 GS.OK027 N4.T35B N4.Z38B NM.UALR OK.BCOK
OK.U32A OK.X37A TA.TUL1 TA.W39A TA.X40A US.KSU1 US.MIAR
US.WMOK
Filtering commands used:
cut a -30 a 180
rtr
taper w 0.1
hp c 0.02 n 3
lp c 0.10 n 3
br c 0.12 0.25 n 4 p 2
Best Fitting Double Couple
Mo = 3.76e+21 dyne-cm
Mw = 3.65
Z = 6 km
Plane Strike Dip Rake
NP1 110 80 -35
NP2 207 56 -168
Principal Axes:
Axis Value Plunge Azimuth
T 3.76e+21 16 163
N 0.00e+00 54 276
P -3.76e+21 31 63
Moment Tensor: (dyne-cm)
Component Value
Mxx 2.60e+21
Mxy -2.09e+21
Mxz -1.72e+21
Myy -1.86e+21
Myz -1.20e+21
Mzz -7.37e+20
##############
#################-----
################------------
###############---------------
###############-------------------
##############----------------------
##############---------------- -----
-#############----------------- P ------
----#########------------------ ------
--------#####-----------------------------
------------------------------------------
-----------#####--------------------------
-----------##########---------------------
---------#################--------------
---------#########################------
--------##############################
-------#############################
------############################
----##########################
---############### #######
-############## T ####
###########
Global CMT Convention Moment Tensor:
R T P
-7.37e+20 -1.72e+21 1.20e+21
-1.72e+21 2.60e+21 2.09e+21
1.20e+21 2.09e+21 -1.86e+21
Details of the solution is found at
http://www.eas.slu.edu/eqc/eqc_mt/MECH.NA/20140420190713/index.html
|
STK = 110
DIP = 80
RAKE = -35
MW = 3.65
HS = 6.0
The NDK file is 20140420190713.ndk The waveform inversion is preferred.
Given the availability of digital waveforms for determination of the moment tensor, this section documents the added processing leading to mLg, if appropriate to the region, and ML by application of the respective IASPEI formulae. As a research study, the linear distance term of the IASPEI formula for ML is adjusted to remove a linear distance trend in residuals to give a regionally defined ML. The defined ML uses horizontal component recordings, but the same procedure is applied to the vertical components since there may be some interest in vertical component ground motions. Residual plots versus distance may indicate interesting features of ground motion scaling in some distance ranges. A residual plot of the regionalized magnitude is given as a function of distance and azimuth, since data sets may transcend different wave propagation provinces.

Left: mLg computed using the IASPEI formula. Center: mLg residuals versus epicentral distance ; the values used for the trimmed mean magnitude estimate are indicated.
Right: residuals as a function of distance and azimuth.
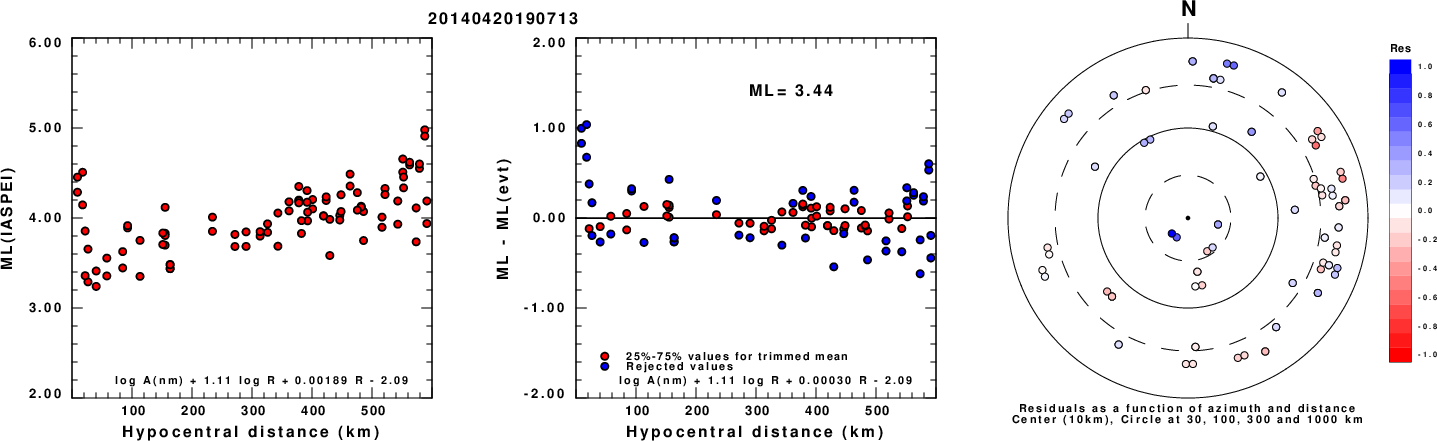
Left: ML computed using the IASPEI formula for Horizontal components. Center: ML residuals computed using a modified IASPEI formula that accounts for path specific attenuation; the values used for the trimmed mean are indicated. The ML relation used for each figure is given at the bottom of each plot.
Right: Residuals from new relation as a function of distance and azimuth.
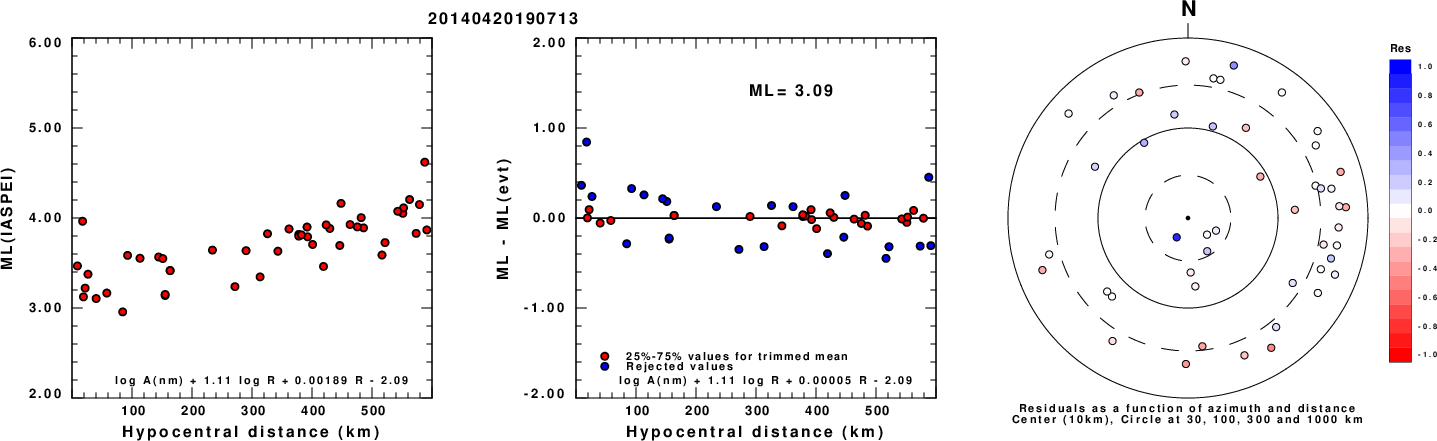
Left: ML computed using the IASPEI formula for Vertical components (research). Center: ML residuals computed using a modified IASPEI formula that accounts for path specific attenuation; the values used for the trimmed mean are indicated. The ML relation used for each figure is given at the bottom of each plot.
Right: Residuals from new relation as a function of distance and azimuth.
 |
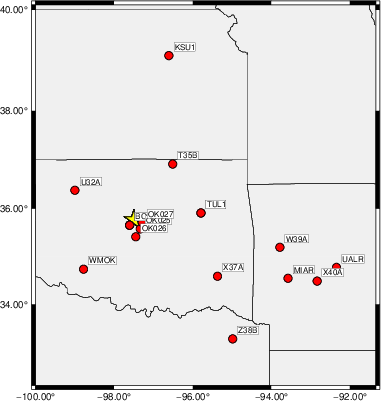
The focal mechanism was determined using broadband seismic waveforms. The location of the event (star) and the stations used for (red) the waveform inversion are shown in the next figure.
|
|
|
The program wvfgrd96 was used with good traces observed at short distance to determine the focal mechanism, depth and seismic moment. This technique requires a high quality signal and well determined velocity model for the Green's functions. To the extent that these are the quality data, this type of mechanism should be preferred over the radiation pattern technique which requires the separate step of defining the pressure and tension quadrants and the correct strike.
The observed and predicted traces are filtered using the following gsac commands:
cut a -30 a 180 rtr taper w 0.1 hp c 0.02 n 3 lp c 0.10 n 3 br c 0.12 0.25 n 4 p 2The results of this grid search are as follow:
DEPTH STK DIP RAKE MW FIT
WVFGRD96 1.0 70 75 -5 3.45 0.3544
WVFGRD96 2.0 125 35 45 3.61 0.5075
WVFGRD96 3.0 110 40 20 3.64 0.5747
WVFGRD96 4.0 110 40 15 3.68 0.6103
WVFGRD96 5.0 300 85 45 3.65 0.6338
WVFGRD96 6.0 110 80 -35 3.65 0.6404
WVFGRD96 7.0 110 75 -30 3.67 0.6403
WVFGRD96 8.0 320 65 60 3.78 0.6282
WVFGRD96 9.0 105 70 -30 3.71 0.6165
WVFGRD96 10.0 110 75 -30 3.72 0.6048
WVFGRD96 11.0 110 75 -30 3.74 0.5942
WVFGRD96 12.0 110 70 -30 3.75 0.5825
WVFGRD96 13.0 110 70 -30 3.76 0.5725
WVFGRD96 14.0 110 65 -30 3.78 0.5613
WVFGRD96 15.0 110 65 -30 3.79 0.5511
WVFGRD96 16.0 110 65 -30 3.80 0.5411
WVFGRD96 17.0 110 65 -30 3.82 0.5288
WVFGRD96 18.0 110 60 -30 3.83 0.5177
WVFGRD96 19.0 110 60 -30 3.84 0.5053
WVFGRD96 20.0 110 60 -30 3.85 0.4929
WVFGRD96 21.0 115 60 -35 3.87 0.4796
WVFGRD96 22.0 115 60 -35 3.88 0.4690
WVFGRD96 23.0 125 60 -35 3.92 0.4578
WVFGRD96 24.0 125 60 -35 3.93 0.4485
WVFGRD96 25.0 125 60 -35 3.93 0.4398
WVFGRD96 26.0 125 55 -40 3.94 0.4308
WVFGRD96 27.0 125 50 -40 3.95 0.4259
WVFGRD96 28.0 150 75 -45 4.01 0.4245
WVFGRD96 29.0 150 70 -45 4.02 0.4237
The best solution is
WVFGRD96 6.0 110 80 -35 3.65 0.6404
The mechanism corresponding to the best fit is
|
|
|
The best fit as a function of depth is given in the following figure:
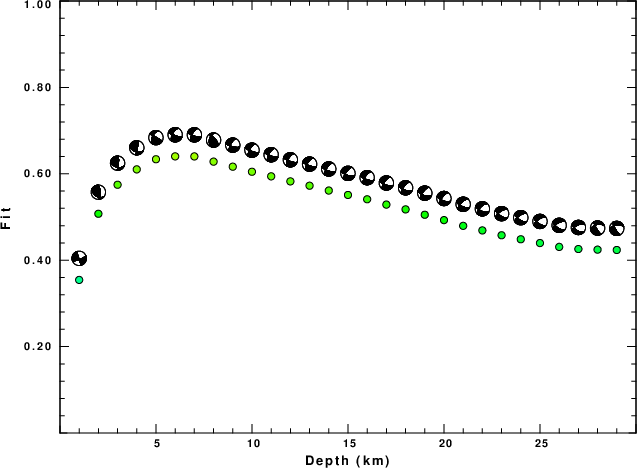
|
|
|
The comparison of the observed and predicted waveforms is given in the next figure. The red traces are the observed and the blue are the predicted. Each observed-predicted component is plotted to the same scale and peak amplitudes are indicated by the numbers to the left of each trace. A pair of numbers is given in black at the right of each predicted traces. The upper number it the time shift required for maximum correlation between the observed and predicted traces. This time shift is required because the synthetics are not computed at exactly the same distance as the observed, the velocity model used in the predictions may not be perfect and the epicentral parameters may be be off. A positive time shift indicates that the prediction is too fast and should be delayed to match the observed trace (shift to the right in this figure). A negative value indicates that the prediction is too slow. The lower number gives the percentage of variance reduction to characterize the individual goodness of fit (100% indicates a perfect fit).
The bandpass filter used in the processing and for the display was
cut a -30 a 180 rtr taper w 0.1 hp c 0.02 n 3 lp c 0.10 n 3 br c 0.12 0.25 n 4 p 2
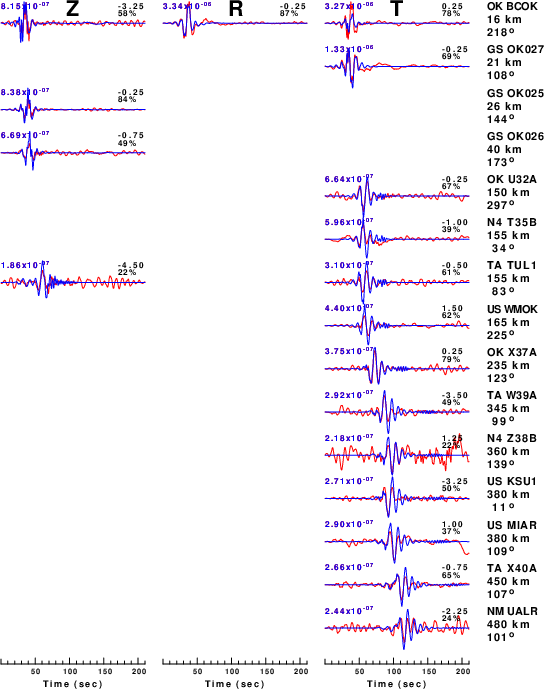
|
| Figure 3. Waveform comparison for selected depth. Red: observed; Blue - predicted. The time shift with respect to the model prediction is indicated. The percent of fit is also indicated. The time scale is relative to the first trace sample. |
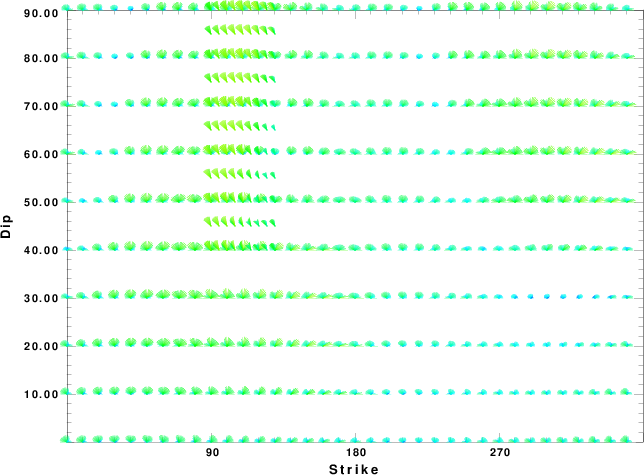
|
| Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to the waveforms. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure. |
A check on the assumed source location is possible by looking at the time shifts between the observed and predicted traces. The time shifts for waveform matching arise for several reasons:
Time_shift = A + B cos Azimuth + C Sin Azimuth
The time shifts for this inversion lead to the next figure:
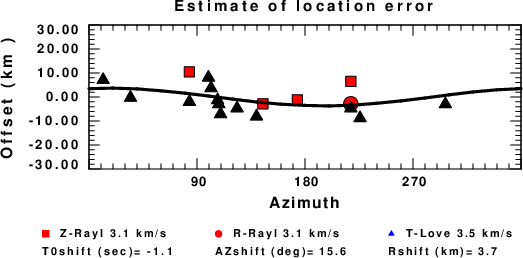
The derived shift in origin time and epicentral coordinates are given at the bottom of the figure.
The WUS.model used for the waveform synthetic seismograms and for the surface wave eigenfunctions and dispersion is as follows (The format is in the model96 format of Computer Programs in Seismology).
MODEL.01
Model after 8 iterations
ISOTROPIC
KGS
FLAT EARTH
1-D
CONSTANT VELOCITY
LINE08
LINE09
LINE10
LINE11
H(KM) VP(KM/S) VS(KM/S) RHO(GM/CC) QP QS ETAP ETAS FREFP FREFS
1.9000 3.4065 2.0089 2.2150 0.302E-02 0.679E-02 0.00 0.00 1.00 1.00
6.1000 5.5445 3.2953 2.6089 0.349E-02 0.784E-02 0.00 0.00 1.00 1.00
13.0000 6.2708 3.7396 2.7812 0.212E-02 0.476E-02 0.00 0.00 1.00 1.00
19.0000 6.4075 3.7680 2.8223 0.111E-02 0.249E-02 0.00 0.00 1.00 1.00
0.0000 7.9000 4.6200 3.2760 0.164E-10 0.370E-10 0.00 0.00 1.00 1.00