2004/12/16 18:59:14 41.79N 127.94E 8 3.97 Korea
SLU Moment Tensor Solution
2004/12/16 18:59:14 41.79N 127.94E 8 3.97 Korea
Best Fitting Double Couple
Mo = 1.01e+22 dyne-cm
Mw = 3.97
Z = 8 km
Plane Strike Dip Rake
NP1 315 80 -15
NP2 48 75 -170
Principal Axes:
Axis Value Plunge Azimuth
T 1.01e+22 3 2
N 0.00e+00 72 102
P -1.01e+22 18 271
Moment Tensor: (dyne-cm)
Component Value
Mxx 1.01e+22
Mxy 4.48e+20
Mxz 5.40e+20
Myy -9.17e+21
Myz 2.94e+21
Mzz -8.95e+20
###### T #####
########## #########
############################
--############################
-------#########################--
----------######################----
--------------#################-------
-----------------##############---------
-------------------###########----------
-- -----------------#######-------------
-- P -------------------###---------------
-- --------------------#----------------
------------------------###---------------
--------------------########------------
------------------###########-----------
---------------###############--------
-----------###################------
-------#######################----
#############################-
############################
######################
##############
Harvard Convention
Moment Tensor:
R T F
-8.95e+20 5.40e+20 -2.94e+21
5.40e+20 1.01e+22 -4.48e+20
-2.94e+21 -4.48e+20 -9.17e+21
|
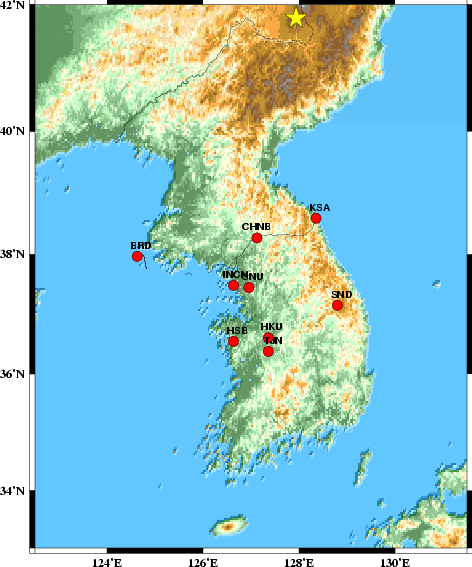
The focal mechanism was determined using broadband seismic waveforms. The location of the event and the station distribution are given in Figure 1.

|
|
|
STK = 315
DIP = 80
RAKE = -15
MW = 3.97
HS = 8
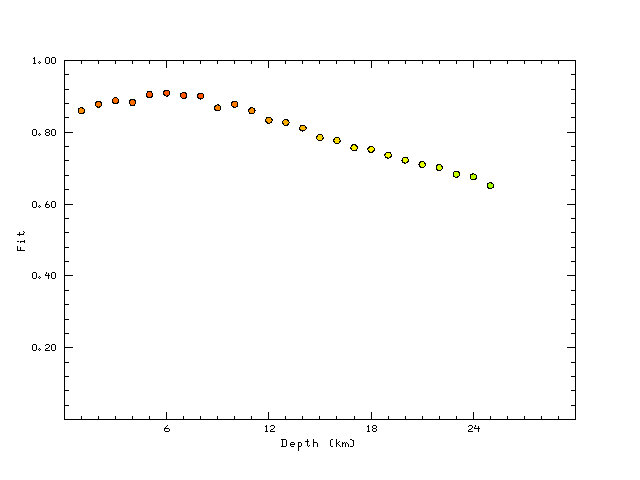
The surface-wave amplitude inversion is preferred. The waveform fit is satisfactory but lacks depth control.
The program wvfgrd96 was used with good traces observed at short distance to determine the focal mechanism, depth and seismic moment. This technique requires a high quality signal and well determined velocity model for the Green functions. To the extent that these are the quality data, this type of mechanism should be preferred over the radiation pattern technique which requires the separate step of defining the pressure and tension quadrants and the correct strike.
The observed and predicted traces are filtered using the following gsac commands:
hp c 0.02 3 lp c 0.10 3 br c 0.13 0.2 n 4 p 2The results of this grid search from 0.5 to 19 km depth are as follow:
DEPTH STK DIP RAKE MW FIT
WVFGRD96 0.5 150 30 40 4.02 0.4306
WVFGRD96 1.0 135 40 30 3.88 0.4167
WVFGRD96 2.0 310 85 10 3.80 0.4532
WVFGRD96 3.0 130 90 -10 3.83 0.4803
WVFGRD96 4.0 315 85 10 3.89 0.4946
WVFGRD96 5.0 315 90 10 3.91 0.5081
WVFGRD96 6.0 315 85 -15 3.93 0.5250
WVFGRD96 7.0 315 85 -15 3.94 0.5305
WVFGRD96 8.0 315 80 -15 3.97 0.5327
WVFGRD96 9.0 315 85 -10 3.96 0.5309
WVFGRD96 10.0 315 85 -10 3.97 0.5227
WVFGRD96 11.0 315 85 -10 3.97 0.5181
WVFGRD96 12.0 315 85 -10 3.98 0.5082
WVFGRD96 13.0 315 85 -5 3.99 0.5023
WVFGRD96 14.0 315 85 -5 4.00 0.4969
WVFGRD96 15.0 135 85 -5 4.00 0.4887
WVFGRD96 16.0 135 85 0 4.01 0.4835
WVFGRD96 17.0 130 85 -5 3.99 0.4781
WVFGRD96 18.0 130 85 -5 4.00 0.4725
WVFGRD96 19.0 130 85 -5 4.01 0.4662
WVFGRD96 20.0 130 85 -5 4.03 0.4592
WVFGRD96 21.0 130 85 -5 4.04 0.4506
WVFGRD96 22.0 310 20 25 4.14 0.4418
WVFGRD96 23.0 310 20 25 4.15 0.4366
WVFGRD96 24.0 310 20 25 4.15 0.4299
WVFGRD96 25.0 310 20 25 4.16 0.4245
The best solution is
WVFGRD96 8.0 315 80 -15 3.97 0.5327
The mechanism correspond to the best fit is
|
|
|
The best fit as a function of depth is given in the following figure:

|
|
|
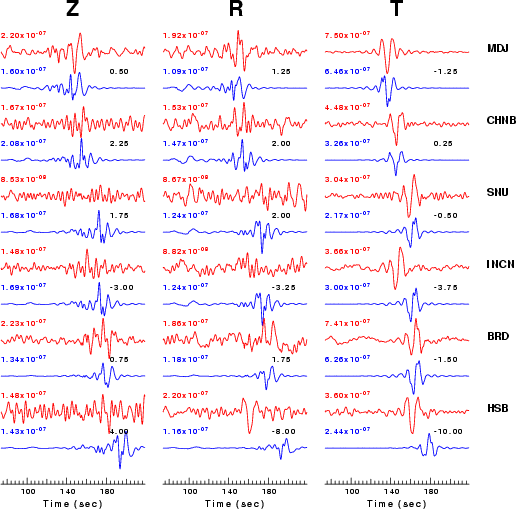
The comparison of the observed and predicted waveforms is given in the next figure. The red traces are the observed and the blue are the predicted. Each observed-predicted componnet is plotted to the same scale and peak amplitudes are indicated by the numbers to the left of each trace. The number in black at the rightr of each predicted traces it the time shift required for maximum correlation between the observed and predicted traces. This time shift is required because the synthetics are not computed at exactly the same distance as the observed and because the velocity model used in the predictions may not be perfect. A positive time shift indicates that the prediction is too fast and should be delayed to match the observed trace (shift to the right in this figure). A negative value indicates that the prediction is too slow. The bandpass filter used in the processing and for the display was
hp c 0.02 3 lp c 0.10 3 br c 0.13 0.2 n 4 p 2
|
|
|
|
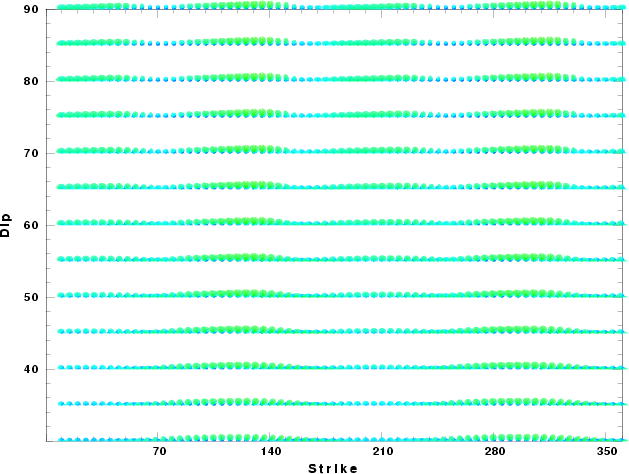
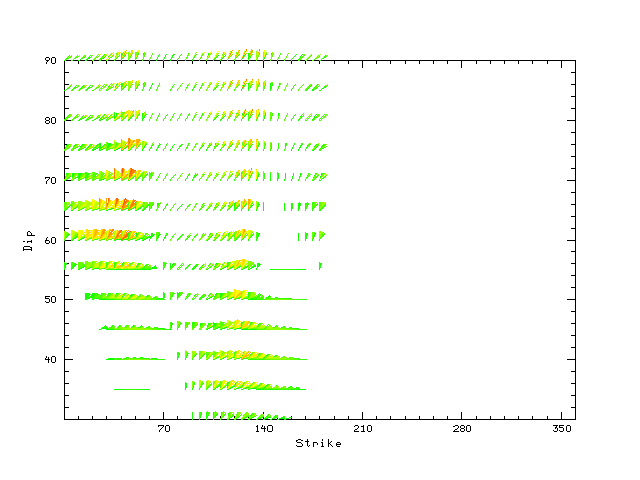
| Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to thewavefroms. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure. |
NODAL PLANES
STK= 308.28
DIP= 85.29
RAKE= -20.07
OR
STK= 40.00
DIP= 70.00
RAKE= -174.99
DEPTH = 6.0 km
Mw = 3.93
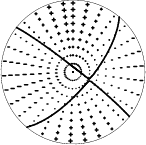
Best Fit 0.9091 - P-T axis plot gives solutions with FIT greater than FIT90
 |
The P-wave first motion data for focal mechanism studies are as follow:
Sta Az(deg) Dist(km) First motion MDJ 23 341 eP_+ CHNB 190 397 eP_- SNU 190 489 eP_X INCN 194 491 eP_+ BRD 215 510 eP_- HSB 191 592 eP_X
Surface wave analysis was performed using codes from Computer Programs in Seismology, specifically the multiple filter analysis program do_mft and the surface-wave radiation pattern search program srfgrd96.
Digital data were collected, instrument response removed and traces converted
to Z, R an T components. Multiple filter analysis was applied to the Z and T traces to obtain the Rayleigh- and Love-wave spectral amplitudes, respectively.
These were input to the search program which examined all depths between 1 and 25 km
and all possible mechanisms.
|
|
|
|
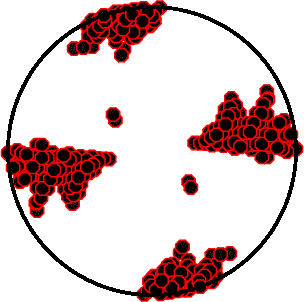
| Pressure-tension axis trends. Since the surface-wave spectra search does not distinguish between P and T axes and since there is a 180 ambiguity in strike, all possible P and T axes are plotted. First motion data and waveforms will be used to select the preferred mechanism. The purpose of this plot is to provide an idea of the possible range of solutions. The P and T-axes for all mechanisms with goodness of fit greater than 0.9 FITMAX (above) are plotted here. |
|
| Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to the Love and Rayleigh wave radiation patterns. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure. Because of the symmetry of the spectral amplitude rediation patterns, only strikes from 0-180 degrees are sampled. |
The distribution of broadband stations with azimuth and distance is
Sta Az(deg) Dist(km) MDJ 23 341 KSA 174 357 CHNB 190 397 SNU 190 489 INCN 194 491 BRD 215 510 SND 172 520 HKU 185 577 HSB 191 592 TJN 185 603 BJT 263 1010
Since the analysis of the surface-wave radiation patterns uses only spectral amplitudes and because the surfave-wave radiation patterns have a 180 degree symmetry, each surface-wave solution consists of four possible focal mechanisms corresponding to the interchange of the P- and T-axes and a roation of the mechanism by 180 degrees. To select one mechanism, P-wave first motion can be used. This was not possible in this case because all the P-wave first motions were emergent ( a feature of the P-wave wave takeoff angle, the station location and the mechanism). The other way to select among the mechanisms is to compute forward synthetics and compare the observed and predicted waveforms.
The fits to the waveforms with the given mechanism are show below:
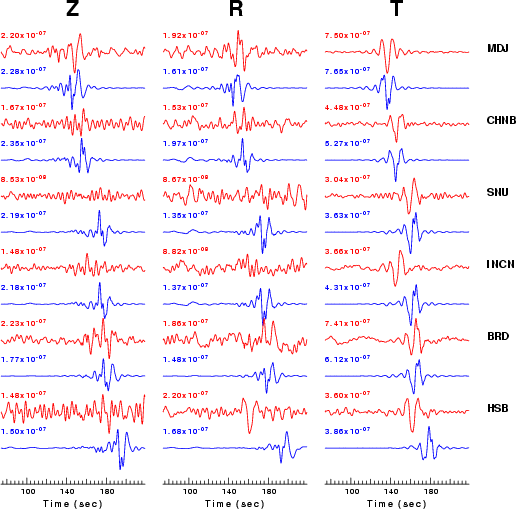
|
This figure shows the fit to the three components of motion (Z - vertical, R-radial and T - transverse). For each station and component, the observed traces is shown in red and the model predicted trace in blue. The traces represent filtered ground velocity in units of meters/sec (the peak value is printed adjacent to each trace; each pair of traces to plotted to the same scale to emphasize the difference in levels). Both synthetic and observed traces have been filtered using the SAC commands:
hp c 0.02 3 lp c 0.10 3 br c 0.13 0.2 n 4 p 2
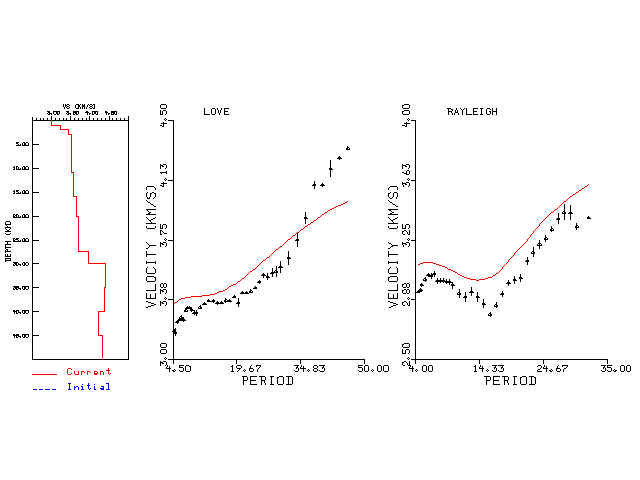
|
|
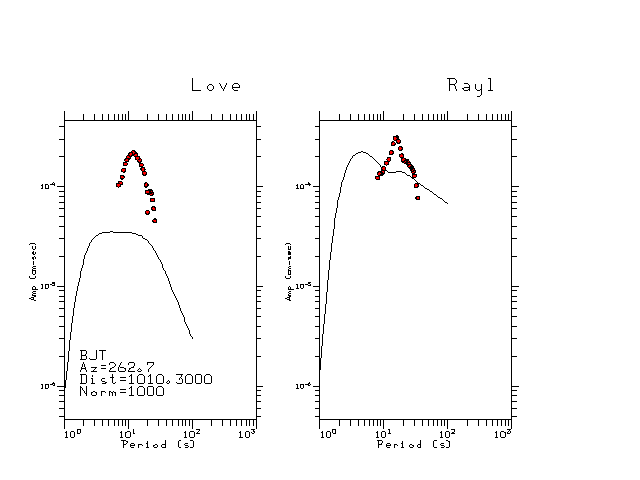
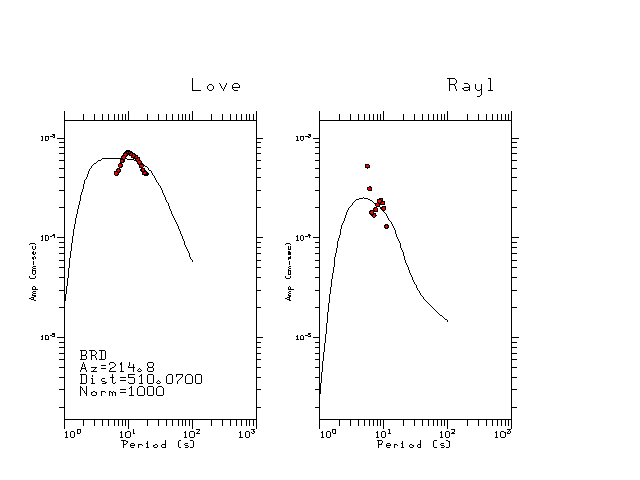
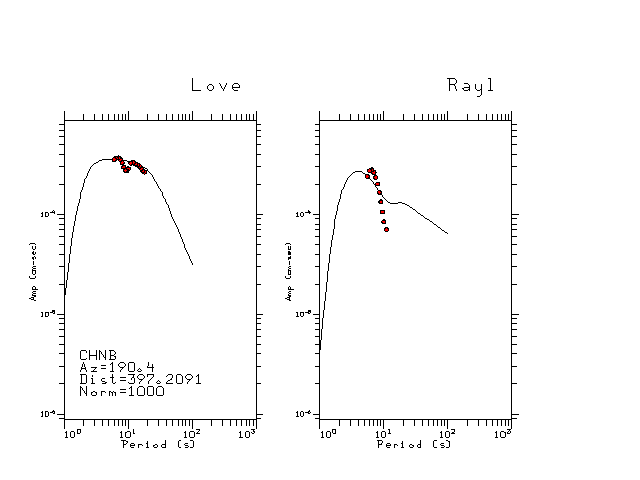
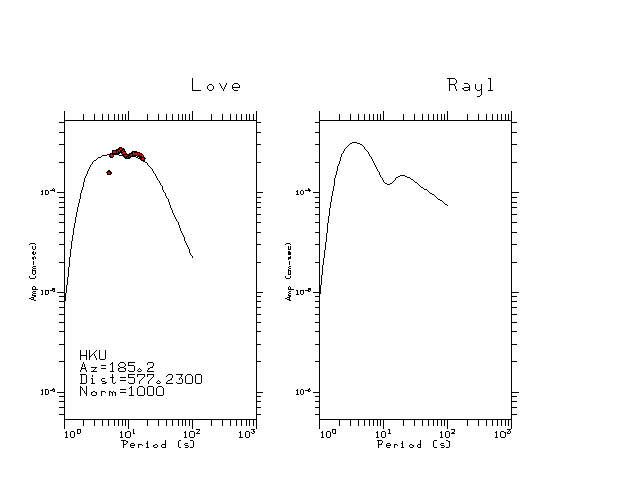
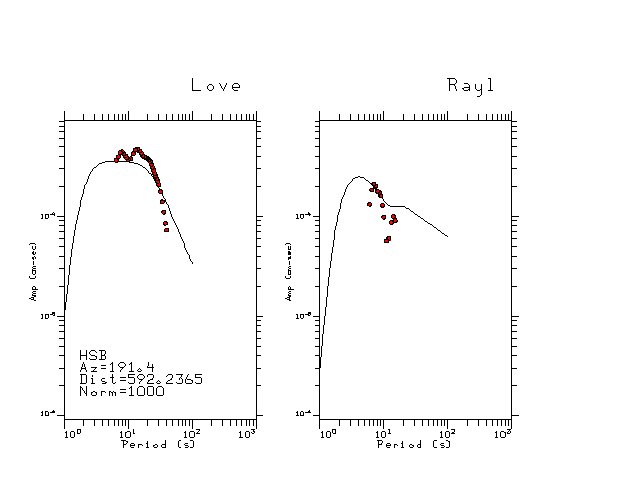
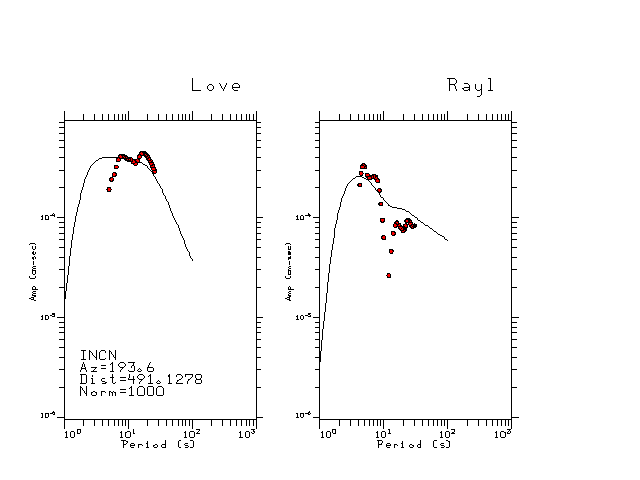
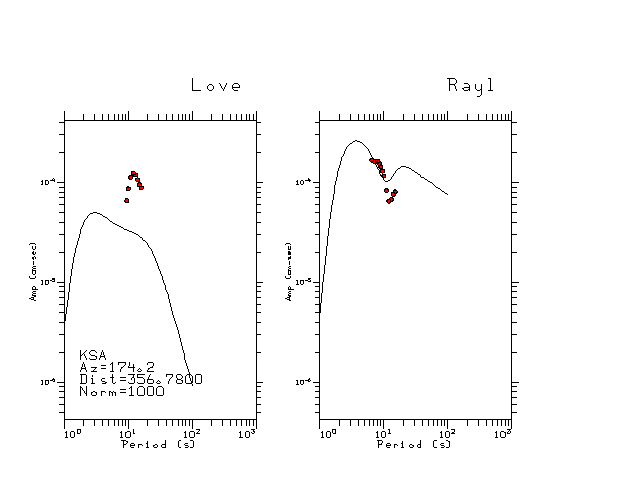
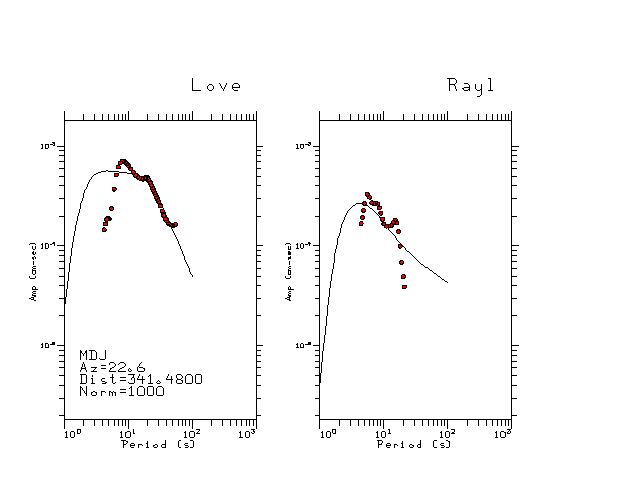
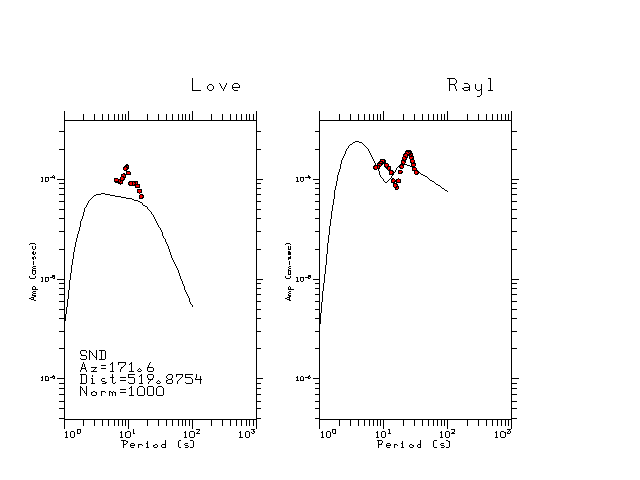
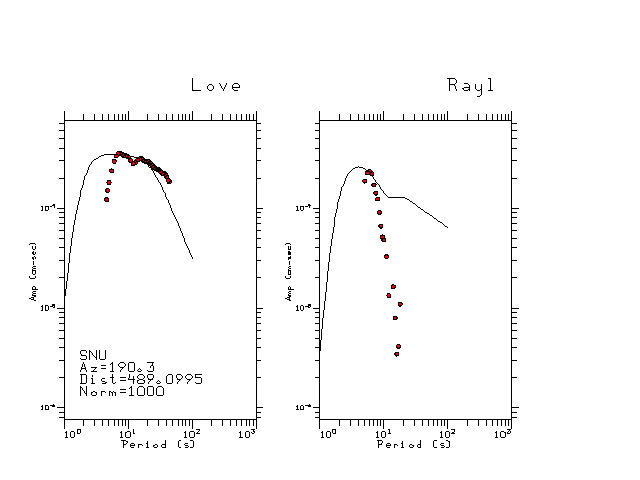
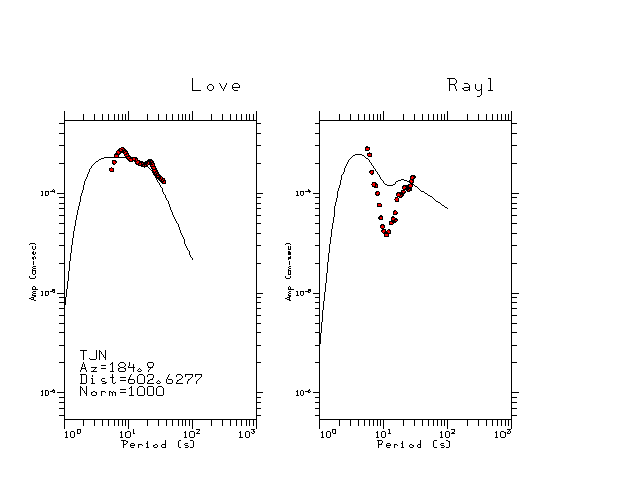
The figures below show the observed spectral amplitudes (units of cm-sec) at each station and the
theoretical predictions as a function of period for the mechanism given above. The modified Utah model earth model
was used to define the Green's functions. For each station, the Love and Rayleigh wave spectrail amplitudes are plotted with the same scaling so that one can get a sense fo the effects of the effects of the focal mechanism and depth on the excitation of each.
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
Here we tabulate the reasons for not using certain digital data sets