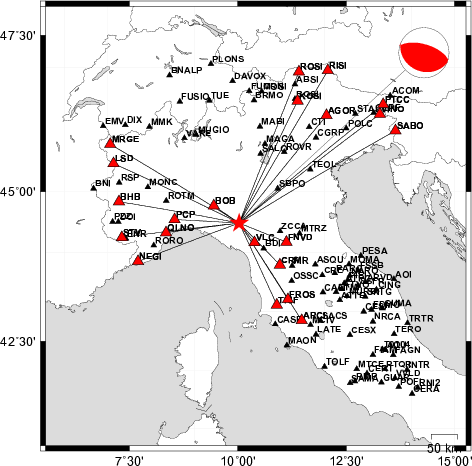
Location
2012/01/27 14:53:13 44.483 10.033 5.4 60.8 Italy
Arrival Times (from USGS)
Arrival time list
Felt Map
USGS Felt map for this earthquake
USGS Felt reports page for
Focal Mechanism
SLU Moment Tensor Solution
ENS 2012/01/27 14:53:13:0 44.48 10.03 5.4 60.8 Italy
Stations used:
GU.BHB GU.FINB GU.NEGI GU.PCP GU.RORO GU.RSP GU.STV GU.TRAV
IV.BOB IV.CASP IV.CRMI IV.DOI IV.FIR IV.FNVD IV.FROS
IV.MABI IV.MCIV IV.MSSA IV.MTRZ IV.PARC IV.PIEI IV.PLMA
IV.PRMA IV.QLNO IV.ROVR IV.TRIF MN.TUE MN.VLC NI.CGRP
Filtering commands used:
hp c 0.02 n 3
lp c 0.06 n 3
Best Fitting Double Couple
Mo = 4.12e+23 dyne-cm
Mw = 5.01
Z = 54 km
Plane Strike Dip Rake
NP1 294 65 88
NP2 120 25 95
Principal Axes:
Axis Value Plunge Azimuth
T 4.12e+23 70 200
N 0.00e+00 2 295
P -4.12e+23 20 26
Moment Tensor: (dyne-cm)
Component Value
Mxx -2.49e+23
Mxy -1.29e+23
Mxz -2.45e+23
Myy -6.55e+22
Myz -1.04e+23
Mzz 3.14e+23
--------------
----------------- --
-------------------- P -----
--------------------- ------
----------------------------------
------------------------------------
-#############------------------------
-####################-------------------
-########################---------------
--###########################-------------
---#############################----------
---###############################--------
----############## ###############------
---############## T #################---
-----############ ##################--
-----#################################
------##############################
-------##########################-
-------######################-
-----------############-----
----------------------
--------------
Global CMT Convention Moment Tensor:
R T P
3.14e+23 -2.45e+23 1.04e+23
-2.45e+23 -2.49e+23 1.29e+23
1.04e+23 1.29e+23 -6.55e+22
Details of the solution is found at
http://www.eas.slu.edu/eqc/eqc_mt/MECH.IT/20120127145313/index.html
|
Preferred Solution
The preferred solution from an analysis of the surface-wave spectral amplitude radiation pattern, waveform inversion and first motion observations is
STK = 120
DIP = 25
RAKE = 95
MW = 5.01
HS = 54.0
The WUS model was used sinc ethe lower crust of nnCIA is not well defined and since I did not have Green functions for the deepter depths requried for this event.
Moment Tensor Comparison
The following compares this source inversion to others
| SLU |
INGVTDMT |
SLU Moment Tensor Solution
ENS 2012/01/27 14:53:13:0 44.48 10.03 5.4 60.8 Italy
Stations used:
GU.BHB GU.FINB GU.NEGI GU.PCP GU.RORO GU.RSP GU.STV GU.TRAV
IV.BOB IV.CASP IV.CRMI IV.DOI IV.FIR IV.FNVD IV.FROS
IV.MABI IV.MCIV IV.MSSA IV.MTRZ IV.PARC IV.PIEI IV.PLMA
IV.PRMA IV.QLNO IV.ROVR IV.TRIF MN.TUE MN.VLC NI.CGRP
Filtering commands used:
hp c 0.02 n 3
lp c 0.06 n 3
Best Fitting Double Couple
Mo = 4.12e+23 dyne-cm
Mw = 5.01
Z = 54 km
Plane Strike Dip Rake
NP1 294 65 88
NP2 120 25 95
Principal Axes:
Axis Value Plunge Azimuth
T 4.12e+23 70 200
N 0.00e+00 2 295
P -4.12e+23 20 26
Moment Tensor: (dyne-cm)
Component Value
Mxx -2.49e+23
Mxy -1.29e+23
Mxz -2.45e+23
Myy -6.55e+22
Myz -1.04e+23
Mzz 3.14e+23
--------------
----------------- --
-------------------- P -----
--------------------- ------
----------------------------------
------------------------------------
-#############------------------------
-####################-------------------
-########################---------------
--###########################-------------
---#############################----------
---###############################--------
----############## ###############------
---############## T #################---
-----############ ##################--
-----#################################
------##############################
-------##########################-
-------######################-
-----------############-----
----------------------
--------------
Global CMT Convention Moment Tensor:
R T P
3.14e+23 -2.45e+23 1.04e+23
-2.45e+23 -2.49e+23 1.29e+23
1.04e+23 1.29e+23 -6.55e+22
Details of the solution is found at
http://www.eas.slu.edu/eqc/eqc_mt/MECH.IT/20120127145313/index.html
|
|
Waveform Inversion
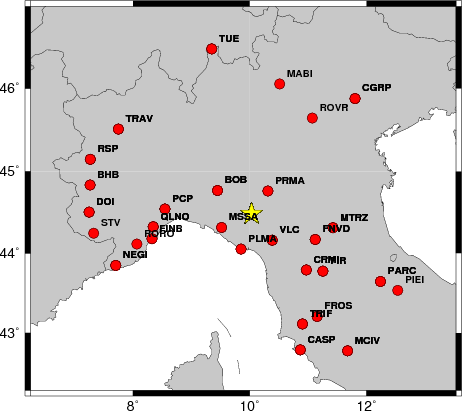
The focal mechanism was determined using broadband seismic waveforms. The location of the event and the
and stations used for the waveform inversion are shown in the next figure.
|
|
Location of broadband stations used for waveform inversion
|
The program wvfgrd96 was used with good traces observed at short distance to determine the focal mechanism, depth and seismic moment. This technique requires a high quality signal and well determined velocity model for the Green functions. To the extent that these are the quality data, this type of mechanism should be preferred over the radiation pattern technique which requires the separate step of defining the pressure and tension quadrants and the correct strike.
The observed and predicted traces are filtered using the following gsac commands:
hp c 0.02 n 3
lp c 0.06 n 3
The results of this grid search from 0.5 to 19 km depth are as follow:
DEPTH STK DIP RAKE MW FIT
WVFGRD96 1.0 295 40 -90 4.26 0.1545
WVFGRD96 2.0 295 40 -90 4.38 0.1999
WVFGRD96 3.0 120 65 -85 4.46 0.2048
WVFGRD96 4.0 290 25 -95 4.48 0.2157
WVFGRD96 5.0 290 25 -100 4.48 0.2238
WVFGRD96 6.0 110 70 -90 4.48 0.2373
WVFGRD96 7.0 115 70 -90 4.49 0.2499
WVFGRD96 8.0 290 20 -95 4.56 0.2664
WVFGRD96 9.0 285 20 -100 4.56 0.2764
WVFGRD96 10.0 285 20 -100 4.56 0.2838
WVFGRD96 11.0 120 70 -80 4.56 0.2898
WVFGRD96 12.0 125 70 -75 4.56 0.2982
WVFGRD96 13.0 125 70 -75 4.57 0.3058
WVFGRD96 14.0 125 70 -75 4.57 0.3127
WVFGRD96 15.0 125 70 -75 4.58 0.3188
WVFGRD96 16.0 125 75 -70 4.58 0.3250
WVFGRD96 17.0 125 75 -70 4.59 0.3311
WVFGRD96 18.0 125 75 -70 4.60 0.3363
WVFGRD96 19.0 125 75 -70 4.61 0.3393
WVFGRD96 20.0 125 75 -70 4.61 0.3424
WVFGRD96 21.0 125 75 -70 4.63 0.3485
WVFGRD96 22.0 125 75 -70 4.64 0.3505
WVFGRD96 23.0 125 75 -70 4.64 0.3499
WVFGRD96 24.0 130 80 -65 4.65 0.3518
WVFGRD96 25.0 130 80 -65 4.66 0.3527
WVFGRD96 26.0 130 80 -65 4.66 0.3528
WVFGRD96 27.0 130 80 -70 4.67 0.3530
WVFGRD96 28.0 40 25 20 4.67 0.3534
WVFGRD96 29.0 45 25 25 4.67 0.3588
WVFGRD96 30.0 130 10 105 4.69 0.3672
WVFGRD96 31.0 295 80 85 4.70 0.3756
WVFGRD96 32.0 295 80 85 4.71 0.3834
WVFGRD96 33.0 295 80 85 4.71 0.3911
WVFGRD96 34.0 295 80 85 4.72 0.3979
WVFGRD96 35.0 295 75 85 4.73 0.4058
WVFGRD96 36.0 295 75 85 4.74 0.4128
WVFGRD96 37.0 125 15 100 4.74 0.4184
WVFGRD96 38.0 295 70 85 4.76 0.4259
WVFGRD96 39.0 295 65 85 4.78 0.4341
WVFGRD96 40.0 125 25 100 4.90 0.4289
WVFGRD96 41.0 125 25 100 4.91 0.4389
WVFGRD96 42.0 120 25 95 4.92 0.4480
WVFGRD96 43.0 295 65 85 4.93 0.4566
WVFGRD96 44.0 120 25 95 4.94 0.4642
WVFGRD96 45.0 120 25 95 4.95 0.4715
WVFGRD96 46.0 295 65 85 4.96 0.4775
WVFGRD96 47.0 295 65 85 4.97 0.4835
WVFGRD96 48.0 120 25 95 4.97 0.4883
WVFGRD96 49.0 120 25 95 4.98 0.4923
WVFGRD96 50.0 295 65 85 4.99 0.4956
WVFGRD96 51.0 295 65 85 4.99 0.4976
WVFGRD96 52.0 120 25 95 5.00 0.4999
WVFGRD96 53.0 120 25 95 5.00 0.5001
WVFGRD96 54.0 120 25 95 5.01 0.5007
WVFGRD96 55.0 120 25 95 5.01 0.4999
WVFGRD96 56.0 295 65 85 5.02 0.4982
WVFGRD96 57.0 295 65 85 5.02 0.4961
WVFGRD96 58.0 295 65 85 5.02 0.4932
WVFGRD96 59.0 295 65 85 5.03 0.4905
WVFGRD96 60.0 295 65 85 5.03 0.4863
WVFGRD96 61.0 295 65 85 5.03 0.4821
WVFGRD96 62.0 295 65 85 5.03 0.4774
WVFGRD96 63.0 295 65 85 5.03 0.4718
WVFGRD96 64.0 295 65 85 5.03 0.4665
WVFGRD96 65.0 290 65 90 5.03 0.4606
WVFGRD96 66.0 290 65 90 5.03 0.4547
WVFGRD96 67.0 290 65 90 5.03 0.4486
WVFGRD96 68.0 290 65 90 5.03 0.4425
WVFGRD96 69.0 290 65 85 5.03 0.4356
The best solution is
WVFGRD96 54.0 120 25 95 5.01 0.5007
The mechanism correspond to the best fit is
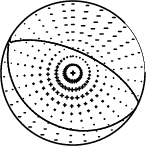
|
|
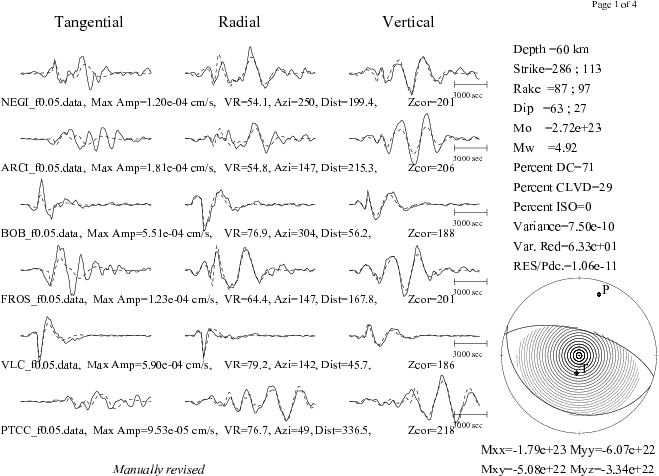
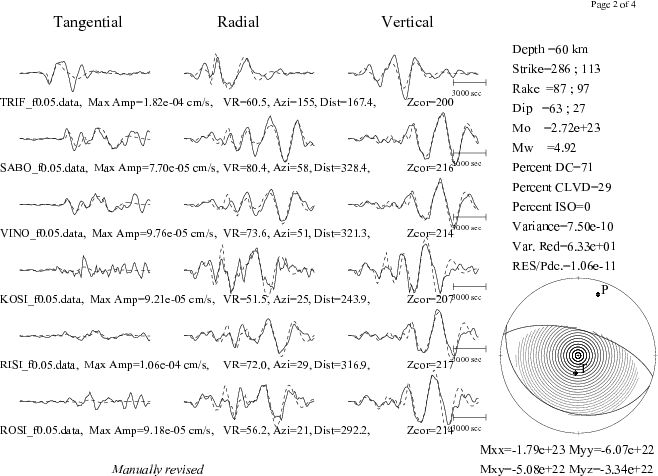
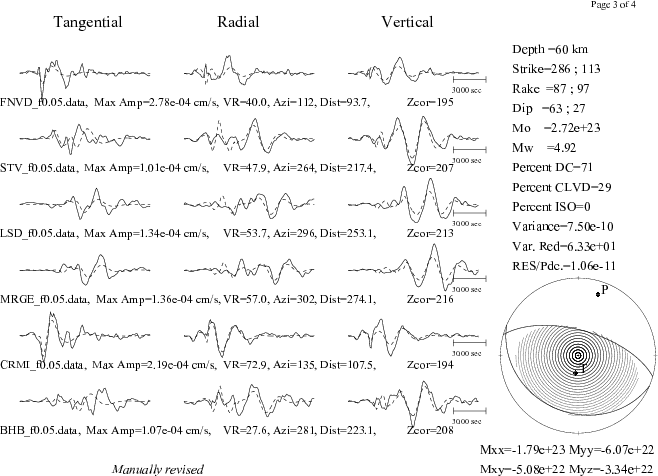
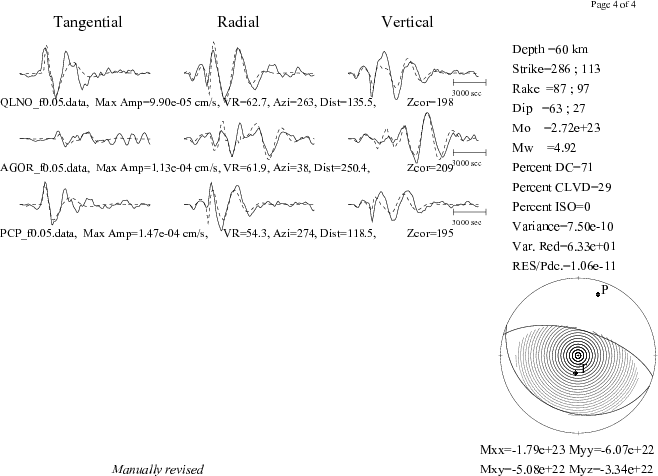
Figure 1. Waveform inversion focal mechanism
|
The best fit as a function of depth is given in the following figure:

|
|
Figure 2. Depth sensitivity for waveform mechanism
|
The comparison of the observed and predicted waveforms is given in the next figure. The red traces are the observed and the blue are the predicted.
Each observed-predicted component is plotted to the same scale and peak amplitudes are indicated by the numbers to the left of each trace. A pair of numbers is given in black at the right of each predicted traces. The upper number it the time shift required for maximum correlation between the observed and predicted traces. This time shift is required because the synthetics are not computed at exactly the same distance as the observed and because the velocity model used in the predictions may not be perfect.
A positive time shift indicates that the prediction is too fast and should be delayed to match the observed trace (shift to the right in this figure). A negative value indicates that the prediction is too slow. The lower number gives the percentage of variance reduction to characterize the individual goodness of fit (100% indicates a perfect fit).
The bandpass filter used in the processing and for the display was
hp c 0.02 n 3
lp c 0.06 n 3

|
|
Figure 3. Waveform comparison for selected depth
|
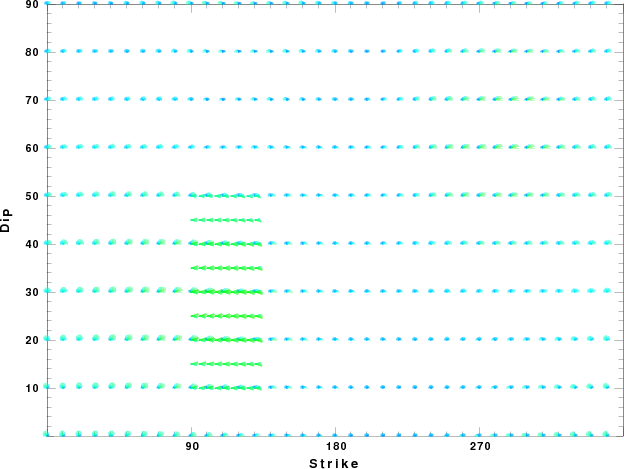
|
|
Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to thewavefroms.
Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure.
|
A check on the assumed source location is possible by looking at the time shifts between the observed and predicted traces. The time shifts for waveform matching arise for several reasons:
- The origin time and epicentral distance are incorrect
- The velocity model used for the inversion is incorrect
- The velocity model used to define the P-arrival time is not the
same as the velocity model used for the waveform inversion
(assuming that the initial trace alignment is based on the
P arrival time)
Assuming only a mislocation, the time shifts are fit to a functional form:
Time_shift = A + B cos Azimuth + C Sin Azimuth
The time shifts for this inversion lead to the next figure:
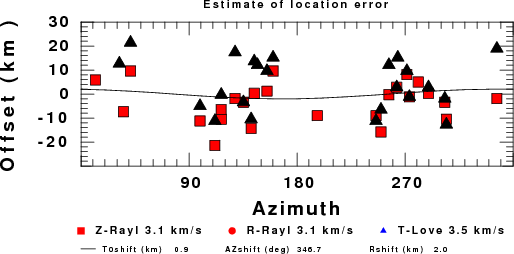
The derived shift in origin time and epicentral coordinates are given at the bottom of the figure.
Discussion
Velocity Model
The WUS used for the waveform synthetic seismograms and for the surface wave eigenfunctions and dispersion is as follows:
MODEL.01
Model after 8 iterations
ISOTROPIC
KGS
FLAT EARTH
1-D
CONSTANT VELOCITY
LINE08
LINE09
LINE10
LINE11
H(KM) VP(KM/S) VS(KM/S) RHO(GM/CC) QP QS ETAP ETAS FREFP FREFS
1.9000 3.4065 2.0089 2.2150 0.302E-02 0.679E-02 0.00 0.00 1.00 1.00
6.1000 5.5445 3.2953 2.6089 0.349E-02 0.784E-02 0.00 0.00 1.00 1.00
13.0000 6.2708 3.7396 2.7812 0.212E-02 0.476E-02 0.00 0.00 1.00 1.00
19.0000 6.4075 3.7680 2.8223 0.111E-02 0.249E-02 0.00 0.00 1.00 1.00
0.0000 7.9000 4.6200 3.2760 0.164E-10 0.370E-10 0.00 0.00 1.00 1.00
Quality Control
Here we tabulate the reasons for not using certain digital data sets
The following stations did not have a valid response files:
DATE=Thu Feb 16 11:22:57 CST 2012
Last Changed 2012/01/27