2008/12/01 10:18:39 35.440 46.109 10.0 5.0 Iran/Iraq Border
2008/12/01 10:18:39 35.440 46.109 10.0 IRAN-IRAQ BORDER REGION M=5.0 (NEIC)
2008/12/01 10:18:41.8 35.45 46.27 15 ML:4.1 18 km East of Marivan, Kordestan Province (IIEES)
Event: 2008-12-01 10:18:38 Lat: 35.3 Lon: 46.2 Depth: 16.0 Mag: 5.0 Type: Mb Origin Author: EMSC-INFO Description: Iran-Iraq border region
USGS Felt map for this earthquake
SLU Moment Tensor Solution
2008/12/01 10:18:39 35.440 46.109 10.0 5.0 Iran/Iraq Border
Best Fitting Double Couple
Mo = 7.85e+22 dyne-cm
Mw = 4.53
Z = 10 km
Plane Strike Dip Rake
NP1 221 87 -50
NP2 315 40 -175
Principal Axes:
Axis Value Plunge Azimuth
T 7.85e+22 30 280
N 0.00e+00 40 38
P -7.85e+22 36 165
Moment Tensor: (dyne-cm)
Component Value
Mxx -4.69e+22
Mxy 3.37e+21
Mxz 4.15e+22
Myy 5.37e+22
Myz -4.32e+22
Mzz -6.74e+21
--------------
----------------------
---#######------------------
#################----------###
######################-----#######
#########################-##########
#########################---##########
#########################------#########
#### ################---------########
##### T ##############------------########
##### #############--------------#######
###################-----------------######
##################------------------######
###############---------------------####
#############-----------------------####
###########------------------------###
########--------------------------##
######------------- -----------#
###-------------- P ----------
---------------- ---------
----------------------
--------------
Harvard Convention
Moment Tensor:
R T F
-6.74e+21 4.15e+22 4.32e+22
4.15e+22 -4.69e+22 -3.37e+21
4.32e+22 -3.37e+21 5.37e+22
Details of the solution is found at
http://www.eas.slu.edu/Earthquake_Center/MECH.IR//index.html
|
STK = 315
DIP = 40
RAKE = -175
MW = 4.53
HS = 10
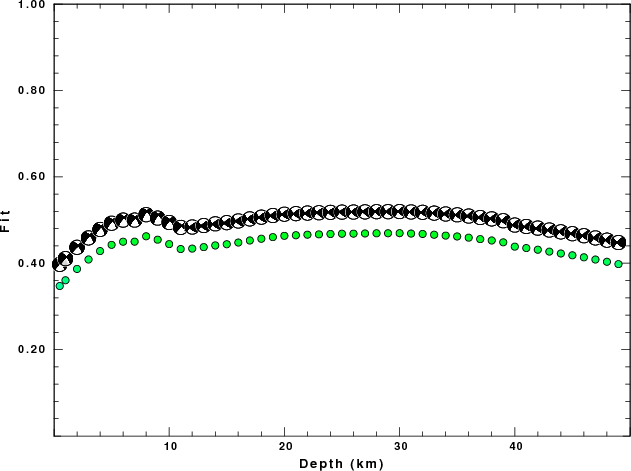
Neither technique provides a good depth constraint. The waveform inversion is affected by the narrow, long period band used for the inversion. The surface-wave technique is affected by the limited azimuthal coverage. However, the gains of the instruments from the different networks seem OK, although there are problems with individual channels. The surface-wave solution is preferred because of the shallower depth.
The focal mechanism was determined using broadband seismic waveforms. The location of the event and the and stations used for the waveform inversion are shown in the next figure.

|
|
|
The program wvfgrd96 was used with good traces observed at short distance to determine the focal mechanism, depth and seismic moment. This technique requires a high quality signal and well determined velocity model for the Green functions. To the extent that these are the quality data, this type of mechanism should be preferred over the radiation pattern technique which requires the separate step of defining the pressure and tension quadrants and the correct strike.
The observed and predicted traces are filtered using the following gsac commands:
hp c 0.016 n 3 lp c 0.03 n 3The results of this grid search from 0.5 to 19 km depth are as follow:
DEPTH STK DIP RAKE MW FIT
WVFGRD96 0.5 225 85 -25 4.25 0.3473
WVFGRD96 1.0 225 85 -25 4.27 0.3606
WVFGRD96 2.0 225 85 -25 4.31 0.3868
WVFGRD96 3.0 220 80 -35 4.37 0.4087
WVFGRD96 4.0 220 75 -35 4.39 0.4282
WVFGRD96 5.0 220 75 -35 4.42 0.4426
WVFGRD96 6.0 220 75 -35 4.43 0.4498
WVFGRD96 7.0 220 75 -30 4.44 0.4499
WVFGRD96 8.0 215 70 -40 4.49 0.4624
WVFGRD96 9.0 215 70 -40 4.50 0.4545
WVFGRD96 10.0 220 75 -30 4.48 0.4443
WVFGRD96 11.0 225 85 -25 4.47 0.4329
WVFGRD96 12.0 230 70 30 4.49 0.4339
WVFGRD96 13.0 230 70 30 4.49 0.4373
WVFGRD96 14.0 230 70 30 4.50 0.4412
WVFGRD96 15.0 230 70 30 4.50 0.4440
WVFGRD96 16.0 230 70 25 4.50 0.4479
WVFGRD96 17.0 230 70 25 4.51 0.4527
WVFGRD96 18.0 230 70 25 4.51 0.4567
WVFGRD96 19.0 230 70 25 4.52 0.4605
WVFGRD96 20.0 230 70 25 4.52 0.4635
WVFGRD96 21.0 230 70 25 4.53 0.4648
WVFGRD96 22.0 230 70 25 4.53 0.4661
WVFGRD96 23.0 230 70 20 4.54 0.4668
WVFGRD96 24.0 230 70 20 4.54 0.4677
WVFGRD96 25.0 230 70 20 4.55 0.4683
WVFGRD96 26.0 230 70 20 4.55 0.4685
WVFGRD96 27.0 230 70 20 4.55 0.4689
WVFGRD96 28.0 225 75 15 4.59 0.4693
WVFGRD96 29.0 225 75 15 4.59 0.4694
WVFGRD96 30.0 225 75 15 4.60 0.4696
WVFGRD96 31.0 225 75 15 4.60 0.4687
WVFGRD96 32.0 225 75 15 4.61 0.4678
WVFGRD96 33.0 225 75 15 4.61 0.4660
WVFGRD96 34.0 225 75 15 4.62 0.4640
WVFGRD96 35.0 225 75 15 4.62 0.4620
WVFGRD96 36.0 225 75 15 4.63 0.4592
WVFGRD96 37.0 225 75 15 4.63 0.4558
WVFGRD96 38.0 225 75 15 4.64 0.4527
WVFGRD96 39.0 225 75 15 4.65 0.4484
WVFGRD96 40.0 225 70 20 4.71 0.4384
WVFGRD96 41.0 225 75 20 4.71 0.4351
WVFGRD96 42.0 225 75 20 4.71 0.4313
WVFGRD96 43.0 225 75 20 4.72 0.4269
WVFGRD96 44.0 225 75 15 4.73 0.4226
WVFGRD96 45.0 225 75 15 4.73 0.4184
WVFGRD96 46.0 225 75 15 4.74 0.4137
WVFGRD96 47.0 225 75 15 4.74 0.4086
WVFGRD96 48.0 225 75 15 4.74 0.4034
WVFGRD96 49.0 225 75 15 4.74 0.3979
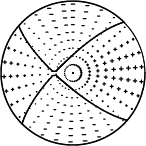
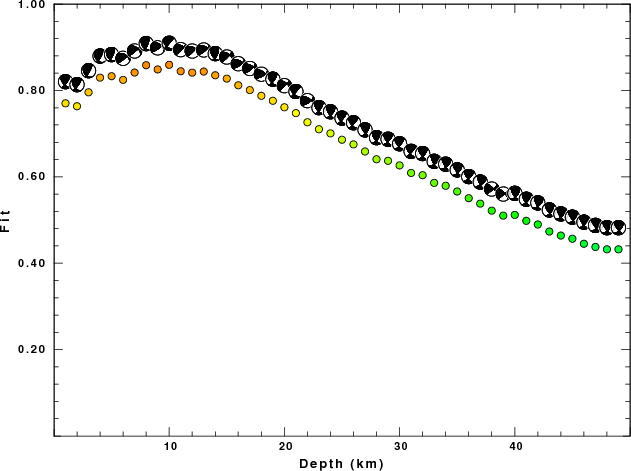
The best solution is
WVFGRD96 30.0 225 75 15 4.60 0.4696
The mechanism correspond to the best fit is
|
|
|
The best fit as a function of depth is given in the following figure:
|
|
|
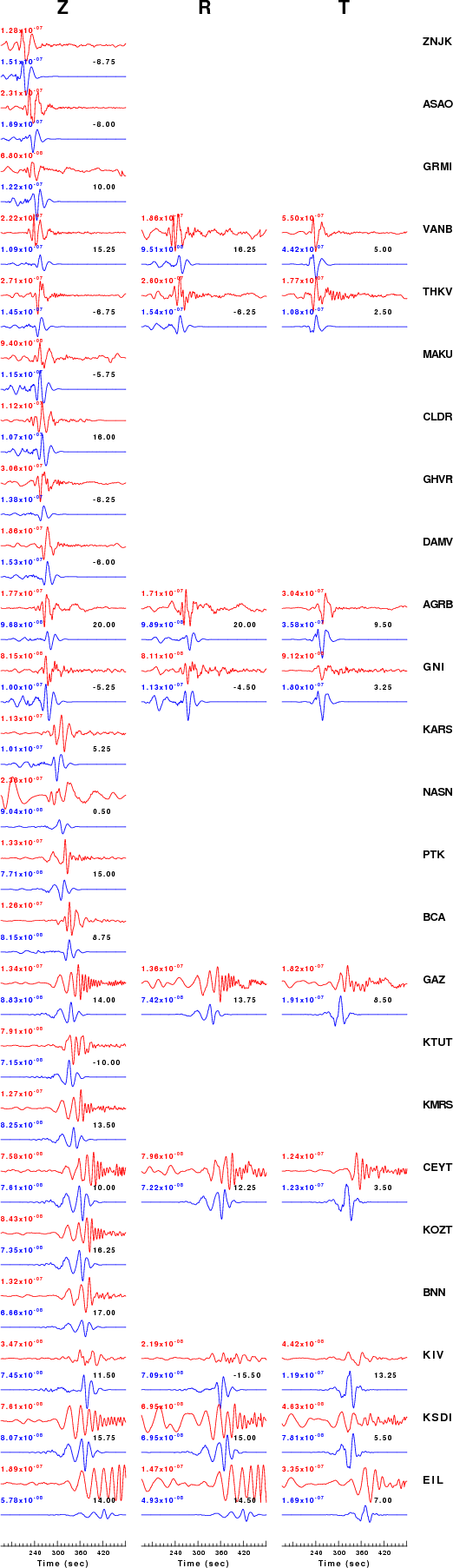
The comparison of the observed and predicted waveforms is given in the next figure. The red traces are the observed and the blue are the predicted. Each observed-predicted componnet is plotted to the same scale and peak amplitudes are indicated by the numbers to the left of each trace. The number in black at the rightr of each predicted traces it the time shift required for maximum correlation between the observed and predicted traces. This time shift is required because the synthetics are not computed at exactly the same distance as the observed and because the velocity model used in the predictions may not be perfect. A positive time shift indicates that the prediction is too fast and should be delayed to match the observed trace (shift to the right in this figure). A negative value indicates that the prediction is too slow. The bandpass filter used in the processing and for the display was
hp c 0.016 n 3 lp c 0.03 n 3
|
|
|
|
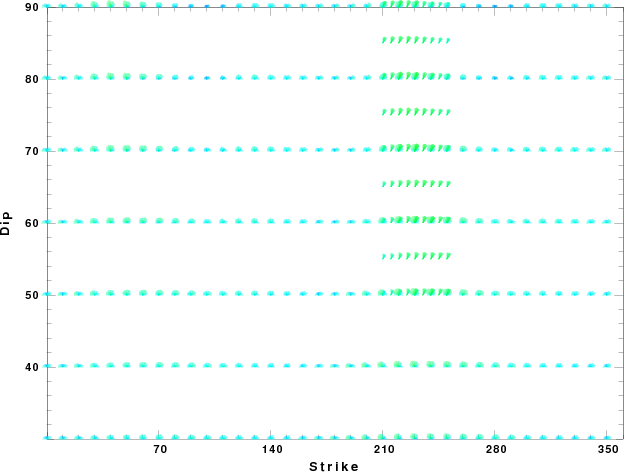
| Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to thewavefroms. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure. |
The following figure shows the stations used in the grid search for the best focal mechanism to fit the surface-wave spectral amplitudes of the Love and Rayleigh waves.

|
|
|
The surface-wave determined focal mechanism is shown here.
NODAL PLANES
STK= 221.14
DIP= 86.78
RAKE= -50.10
OR
STK= 314.98
DIP= 40.01
RAKE= -174.99
DEPTH = 10.0 km
Mw = 4.53
Best Fit 0.8593 - P-T axis plot gives solutions with FIT greater than FIT90
 |
The P-wave first motion data for focal mechanism studies are as follow:
Sta Az(deg) Dist(km) First motion
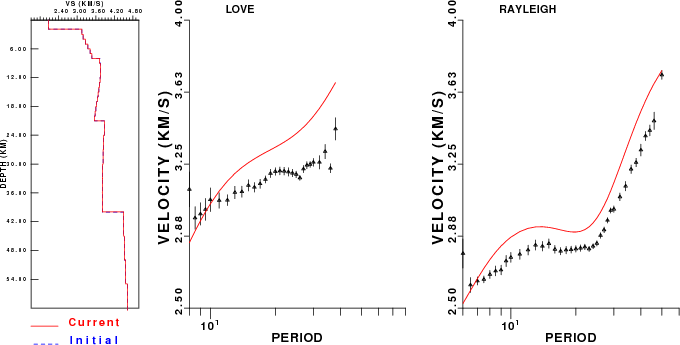
Surface wave analysis was performed using codes from Computer Programs in Seismology, specifically the multiple filter analysis program do_mft and the surface-wave radiation pattern search program srfgrd96.
Digital data were collected, instrument response removed and traces converted
to Z, R an T components. Multiple filter analysis was applied to the Z and T traces to obtain the Rayleigh- and Love-wave spectral amplitudes, respectively.
These were input to the search program which examined all depths between 1 and 25 km
and all possible mechanisms.
|
|
|

|
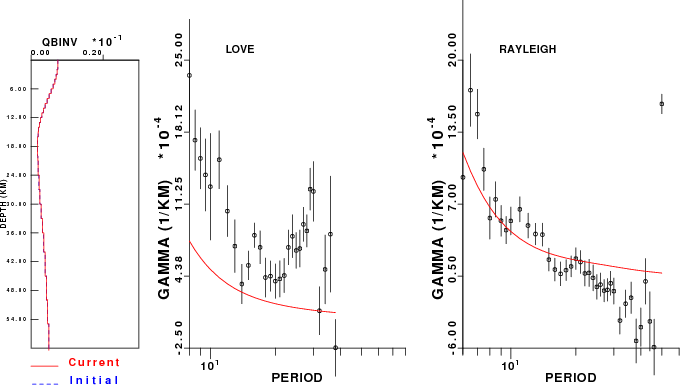
| Pressure-tension axis trends. Since the surface-wave spectra search does not distinguish between P and T axes and since there is a 180 ambiguity in strike, all possible P and T axes are plotted. First motion data and waveforms will be used to select the preferred mechanism. The purpose of this plot is to provide an idea of the possible range of solutions. The P and T-axes for all mechanisms with goodness of fit greater than 0.9 FITMAX (above) are plotted here. |
|
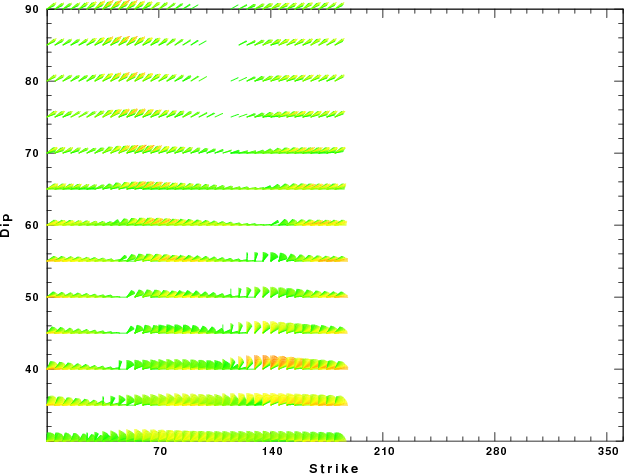
| Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to the Love and Rayleigh wave radiation patterns. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure. Because of the symmetry of the spectral amplitude rediation patterns, only strikes from 0-180 degrees are sampled. |
The distribution of broadband stations with azimuth and distance is
Sta Az(deg) Dist(km) ZNJK 59 269 CUKT 312 301 ASAO 104 371 GRMI 22 406 VANB 326 426 THKV 82 435 MAKU 344 452 CLDR 335 455 CHTH 82 457 GHVR 101 485 DAMV 86 532 AGRB 330 535 GNI 347 536 KARS 336 632 NASN 114 684 PTK 305 709 BCA 331 773 GAZ 286 822 KTUT 320 829 KMRS 288 856 MERS 283 938 CEYT 284 947 KOZT 287 949 BNN 296 986 KIV 344 990 KSDI 258 993 KVT 308 1079 EREN 274 1082 CORM 301 1138 CANT 301 1236 KONT 287 1258 LOD 297 1275 SHUT 289 1425 ELL 280 1464
Since the analysis of the surface-wave radiation patterns uses only spectral amplitudes and because the surfave-wave radiation patterns have a 180 degree symmetry, each surface-wave solution consists of four possible focal mechanisms corresponding to the interchange of the P- and T-axes and a roation of the mechanism by 180 degrees. To select one mechanism, P-wave first motion can be used. This was not possible in this case because all the P-wave first motions were emergent ( a feature of the P-wave wave takeoff angle, the station location and the mechanism). The other way to select among the mechanisms is to compute forward synthetics and compare the observed and predicted waveforms.
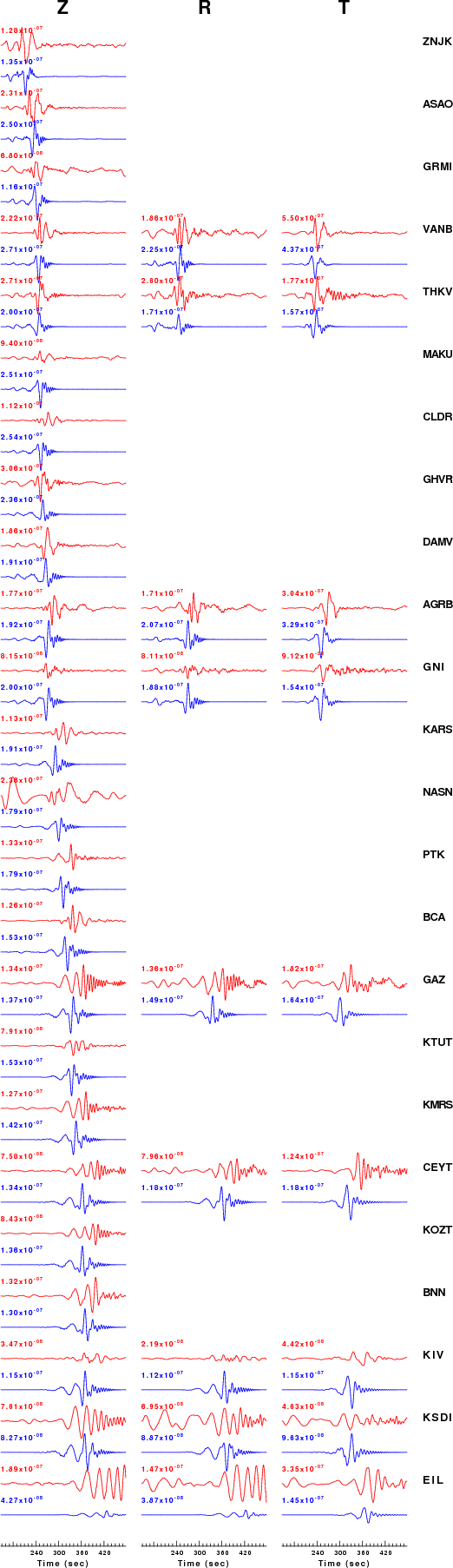
The fits to the waveforms with the given mechanism are show below:
|
This figure shows the fit to the three components of motion (Z - vertical, R-radial and T - transverse). For each station and component, the observed traces is shown in red and the model predicted trace in blue. The traces represent filtered ground velocity in units of meters/sec (the peak value is printed adjacent to each trace; each pair of traces to plotted to the same scale to emphasize the difference in levels). Both synthetic and observed traces have been filtered using the SAC commands:
hp c 0.016 n 3 lp c 0.03 n 3
|
|
The WUS used for the waveform synthetic seismograms and for the surface wave eigenfunctions and dispersion is as follows:
MODEL.01
Model after 8 iterations
ISOTROPIC
KGS
FLAT EARTH
1-D
CONSTANT VELOCITY
LINE08
LINE09
LINE10
LINE11
H(KM) VP(KM/S) VS(KM/S) RHO(GM/CC) QP QS ETAP ETAS FREFP FREFS
1.9000 3.4065 2.0089 2.2150 0.302E-02 0.679E-02 0.00 0.00 1.00 1.00
6.1000 5.5445 3.2953 2.6089 0.349E-02 0.784E-02 0.00 0.00 1.00 1.00
13.0000 6.2708 3.7396 2.7812 0.212E-02 0.476E-02 0.00 0.00 1.00 1.00
19.0000 6.4075 3.7680 2.8223 0.111E-02 0.249E-02 0.00 0.00 1.00 1.00
0.0000 7.9000 4.6200 3.2760 0.164E-10 0.370E-10 0.00 0.00 1.00 1.00
Here we tabulate the reasons for not using certain digital data sets