Location

2014/03/24 11:32:14 -19.833 -70.814 19.8 5.2 Chile

Arrival Times (from USGS)

Felt Map

Focal Mechanism

USGS/SLU Moment Tensor Solution ENS 2014/03/24 11:32:14:0 -19.83 -70.81 19.8 5.2 Chile Stations used: C.GO01 CX.PATCX CX.PB01 CX.PB04 CX.PB07 CX.PB09 CX.PB10 CX.PB11 CX.PB12 CX.PB14 CX.PB15 CX.PB16 CX.PSGCX GT.LPAZ IU.LVC Filtering commands used: cut a -30 a 180 rtr taper w 0.1 hp c 0.02 n 3 lp c 0.06 n 3 Best Fitting Double Couple Mo = 5.43e+23 dyne-cm Mw = 5.09 Z = 22 km Plane Strike Dip Rake NP1 169 71 85 NP2 5 20 105 Principal Axes: Axis Value Plunge Azimuth T 5.43e+23 64 70 N 0.00e+00 5 171 P -5.43e+23 26 263 Moment Tensor: (dyne-cm) Component Value Mxx 5.79e+21 Mxy -1.81e+22 Mxz 9.66e+22 Myy -3.43e+23 Myz 4.12e+23 Mzz 3.37e+23 ---#########-- ------#############--- ---------###############---- ---------##################--- -----------###################---- ------------#####################--- -------------#####################---- --------------######################---- --------------########### ########---- ----------------########## T #########---- ----------------########## #########---- ---- ---------######################---- ---- P ----------#####################---- --- ----------####################---- -----------------###################---- ----------------##################---- ----------------################---- ----------------##############---- --------------############---- --------------##########---- -------------#####---- ----------#--- Global CMT Convention Moment Tensor: R T P 3.37e+23 9.66e+22 -4.12e+23 9.66e+22 5.79e+21 1.81e+22 -4.12e+23 1.81e+22 -3.43e+23 Details of the solution is found at http://www.eas.slu.edu/eqc/eqc_mt/MECH.NA/20140324113214/index.html

Preferred Solution

The preferred solution from an analysis of the surface-wave spectral amplitude radiation pattern, waveform inversion and first motion observations is

      STK = 365
      DIP = 20
     RAKE = 105
       MW = 5.09
       HS = 22.0

The NDK file is 20140324113214.ndk The waveform inversion is preferred.

Moment Tensor Comparison

The following compares this source inversion to others

SLU

USGS/SLU Moment Tensor Solution ENS 2014/03/24 11:32:14:0 -19.83 -70.81 19.8 5.2 Chile Stations used: C.GO01 CX.PATCX CX.PB01 CX.PB04 CX.PB07 CX.PB09 CX.PB10 CX.PB11 CX.PB12 CX.PB14 CX.PB15 CX.PB16 CX.PSGCX GT.LPAZ IU.LVC Filtering commands used: cut a -30 a 180 rtr taper w 0.1 hp c 0.02 n 3 lp c 0.06 n 3 Best Fitting Double Couple Mo = 5.43e+23 dyne-cm Mw = 5.09 Z = 22 km Plane Strike Dip Rake NP1 169 71 85 NP2 5 20 105 Principal Axes: Axis Value Plunge Azimuth T 5.43e+23 64 70 N 0.00e+00 5 171 P -5.43e+23 26 263 Moment Tensor: (dyne-cm) Component Value Mxx 5.79e+21 Mxy -1.81e+22 Mxz 9.66e+22 Myy -3.43e+23 Myz 4.12e+23 Mzz 3.37e+23 ---#########-- ------#############--- ---------###############---- ---------##################--- -----------###################---- ------------#####################--- -------------#####################---- --------------######################---- --------------########### ########---- ----------------########## T #########---- ----------------########## #########---- ---- ---------######################---- ---- P ----------#####################---- --- ----------####################---- -----------------###################---- ----------------##################---- ----------------################---- ----------------##############---- --------------############---- --------------##########---- -------------#####---- ----------#--- Global CMT Convention Moment Tensor: R T P 3.37e+23 9.66e+22 -4.12e+23 9.66e+22 5.79e+21 1.81e+22 -4.12e+23 1.81e+22 -3.43e+23 Details of the solution is found at http://www.eas.slu.edu/eqc/eqc_mt/MECH.NA/20140324113214/index.html

Waveform Inversion

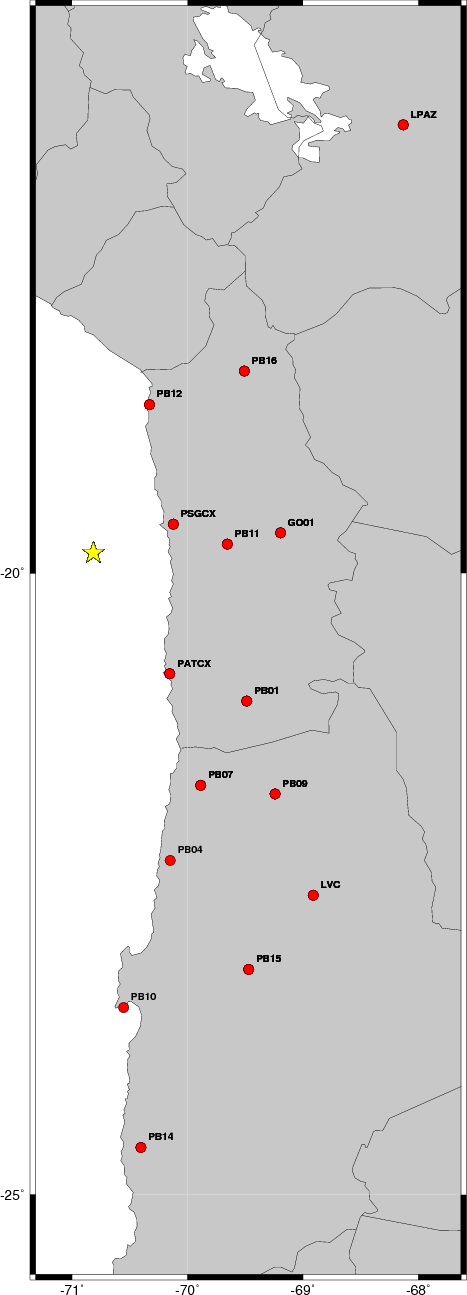
The focal mechanism was determined using broadband seismic waveforms. The location of the event and the and stations used for the waveform inversion are shown in the next figure.

Location of broadband stations used for waveform inversion

The program wvfgrd96 was used with good traces observed at short distance to determine the focal mechanism, depth and seismic moment. This technique requires a high quality signal and well determined velocity model for the Green functions. To the extent that these are the quality data, this type of mechanism should be preferred over the radiation pattern technique which requires the separate step of defining the pressure and tension quadrants and the correct strike.

The observed and predicted traces are filtered using the following gsac commands:

cut a -30 a 180
rtr
taper w 0.1
hp c 0.02 n 3 
lp c 0.06 n 3

The results of this grid search from 0.5 to 19 km depth are as follow:

           DEPTH  STK   DIP  RAKE   MW    FIT
WVFGRD96    2.0   350    50    90   4.81 0.3668
WVFGRD96    4.0   240     5   -20   4.89 0.2664
WVFGRD96    6.0   270     5    10   4.89 0.4115
WVFGRD96    8.0   285     5    25   4.98 0.5029
WVFGRD96   10.0   305    10    45   4.99 0.5972
WVFGRD96   12.0   315    15    55   5.01 0.6707
WVFGRD96   14.0   170    75    90   5.03 0.7315
WVFGRD96   16.0   170    70    90   5.05 0.7757
WVFGRD96   18.0   350    20    90   5.06 0.8023
WVFGRD96   20.0   170    70    90   5.07 0.8154
WVFGRD96   22.0   365    20   105   5.09 0.8177
WVFGRD96   24.0   345    20    85   5.10 0.8116
WVFGRD96   26.0   320    20    65   5.11 0.7993
WVFGRD96   28.0   320    20    65   5.13 0.7820
WVFGRD96   30.0   320    20    65   5.14 0.7593
WVFGRD96   32.0   320    20    65   5.14 0.7320
WVFGRD96   34.0   355    20   100   5.15 0.7014
WVFGRD96   36.0   315    20    60   5.16 0.6701
WVFGRD96   38.0   315    20    60   5.16 0.6401
WVFGRD96   40.0   320    15    65   5.30 0.6145
WVFGRD96   42.0   320    15    65   5.30 0.5800
WVFGRD96   44.0   320    15    65   5.31 0.5473
WVFGRD96   46.0   315    15    60   5.31 0.5167
WVFGRD96   48.0   310    15    55   5.31 0.4882
WVFGRD96   50.0   165    75    90   5.32 0.4637
WVFGRD96   52.0   345    15    90   5.33 0.4409
WVFGRD96   54.0   165    75    90   5.34 0.4204
WVFGRD96   56.0   165    75    90   5.34 0.4019
WVFGRD96   58.0   165    75    90   5.35 0.3848
WVFGRD96   60.0   350    10    95   5.35 0.3697
WVFGRD96   62.0   350    10    95   5.35 0.3567
WVFGRD96   64.0   345    10    90   5.36 0.3444
WVFGRD96   66.0   335     5    80   5.36 0.3338
WVFGRD96   68.0   300     5    40   5.36 0.3243
WVFGRD96   70.0   165    85    90   5.37 0.3162
WVFGRD96   72.0   165    90    90   5.37 0.3099
WVFGRD96   74.0   230     5   -25   5.37 0.3042
WVFGRD96   76.0   310    70   -70   5.36 0.2997
WVFGRD96   78.0   325    70   -80   5.36 0.3027
WVFGRD96   80.0   330    70   -80   5.36 0.3076
WVFGRD96   82.0   330    70   -80   5.37 0.3115
WVFGRD96   84.0   320    60   -90   5.38 0.3170
WVFGRD96   86.0   320    60   -90   5.38 0.3251
WVFGRD96   88.0   140    30   -90   5.39 0.3320
WVFGRD96   90.0   140    30   -90   5.39 0.3392
WVFGRD96   92.0   140    30   -90   5.40 0.3455
WVFGRD96   94.0   320    60   -90   5.40 0.3507
WVFGRD96   96.0   140    30   -90   5.41 0.3563
WVFGRD96   98.0   320    60   -90   5.41 0.3605
WVFGRD96  100.0   320    60   -90   5.42 0.3648
WVFGRD96  102.0   140    30   -90   5.42 0.3680
WVFGRD96  104.0   145    30   -85   5.43 0.3732
WVFGRD96  106.0   155    30   -75   5.44 0.3793
WVFGRD96  108.0   145    35   -80   5.44 0.3824

The best solution is

WVFGRD96   22.0   365    20   105   5.09 0.8177

The mechanism correspond to the best fit is

Figure 1. Waveform inversion focal mechanism

The best fit as a function of depth is given in the following figure:

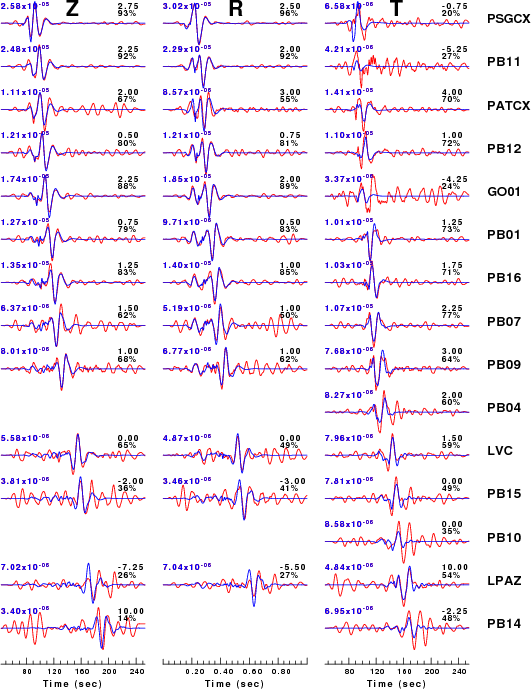
Figure 2. Depth sensitivity for waveform mechanism

The comparison of the observed and predicted waveforms is given in the next figure. The red traces are the observed and the blue are the predicted. Each observed-predicted component is plotted to the same scale and peak amplitudes are indicated by the numbers to the left of each trace. A pair of numbers is given in black at the right of each predicted traces. The upper number it the time shift required for maximum correlation between the observed and predicted traces. This time shift is required because the synthetics are not computed at exactly the same distance as the observed and because the velocity model used in the predictions may not be perfect. A positive time shift indicates that the prediction is too fast and should be delayed to match the observed trace (shift to the right in this figure). A negative value indicates that the prediction is too slow. The lower number gives the percentage of variance reduction to characterize the individual goodness of fit (100% indicates a perfect fit).

The bandpass filter used in the processing and for the display was

cut a -30 a 180
rtr
taper w 0.1
hp c 0.02 n 3 
lp c 0.06 n 3

Figure 3. Waveform comparison for selected depth. Red: observed; Blue - predicted. The time shift with respect to the model prediction is indicated. The percent of fit is also indicated.

Focal mechanism sensitivity at the preferred depth. The red color indicates a very good fit to thewavefroms. Each solution is plotted as a vector at a given value of strike and dip with the angle of the vector representing the rake angle, measured, with respect to the upward vertical (N) in the figure.

A check on the assumed source location is possible by looking at the time shifts between the observed and predicted traces. The time shifts for waveform matching arise for several reasons:

The origin time and epicentral distance are incorrect
The velocity model used for the inversion is incorrect
The velocity model used to define the P-arrival time is not the same as the velocity model used for the waveform inversion (assuming that the initial trace alignment is based on the P arrival time)

Assuming only a mislocation, the time shifts are fit to a functional form:

 Time_shift = A + B cos Azimuth + C Sin Azimuth

The time shifts for this inversion lead to the next figure:

The derived shift in origin time and epicentral coordinates are given at the bottom of the figure.

Discussion

Acknowledgements

Thanks also to the many seismic network operators whose dedication make this effort possible: University of Nevada Reno, University of Alaska, University of Washington, Oregon State University, University of Utah, Montana Bureas of Mines, UC Berkely, Caltech, UC San Diego, Saint Louis University, University of Memphis, Lamont Doherty Earth Observatory, the Iris stations and the Transportable Array of EarthScope.

Velocity Model

The WUS used for the waveform synthetic seismograms and for the surface wave eigenfunctions and dispersion is as follows:

MODEL.01
Model after     8 iterations
ISOTROPIC
KGS
FLAT EARTH
1-D
CONSTANT VELOCITY
LINE08
LINE09
LINE10
LINE11
      H(KM)   VP(KM/S)   VS(KM/S) RHO(GM/CC)         QP         QS       ETAP       ETAS      FREFP      FREFS
     1.9000     3.4065     2.0089     2.2150  0.302E-02  0.679E-02   0.00       0.00       1.00       1.00    
     6.1000     5.5445     3.2953     2.6089  0.349E-02  0.784E-02   0.00       0.00       1.00       1.00    
    13.0000     6.2708     3.7396     2.7812  0.212E-02  0.476E-02   0.00       0.00       1.00       1.00    
    19.0000     6.4075     3.7680     2.8223  0.111E-02  0.249E-02   0.00       0.00       1.00       1.00    
     0.0000     7.9000     4.6200     3.2760  0.164E-10  0.370E-10   0.00       0.00       1.00       1.00

Quality Control

Here we tabulate the reasons for not using certain digital data sets

The following stations did not have a valid response files:

Last Changed Mon Mar 24 11:54:21 CDT 2014